Exercise Zone : Trigonometri

Berikut ini adalah kumpulan soal mengenai Trigonometri tingkat dasar. Jika ada jawaban yang salah, mohon dikoreksi melalui komentar. Terima kasih.

---

No. 1

Sebuah tangga disandarkan pada tembol rumah dengan membentuk sudut 60\degree terhadap tanah. Jarak antara ujung tangga dan permukaan tanah adalah 2\sqrt3 m. Panjang tangga tersebut adalah .....

1. 4 m
2. 4{,}5 m
   
3. 5 m
   

4. 5{,}5 m
5. 6 m
   

dari Yn t di Grup Telegram

\begin{aligned}
\sin60\degree&=\dfrac{2\sqrt3}x\\[8pt]
\dfrac12\sqrt3&=\dfrac{2\sqrt3}x\\[8pt]
x&=\dfrac{2\sqrt3}{\dfrac12\sqrt3}\\
&=\boxed{\boxed{4}}
\end{aligned}

Jadi,

---

No. 2

Jika x-y=\dfrac12\pi maka \tan x adalah....

1. \dfrac{1+\tan y^2}{y}
2. -\dfrac{1-y^2}{\tan y}
   
3. \dfrac{\tan(1-y)}{(1+y)^2}
   

4. \dfrac{\tan y}{(1+y)^2}
5. -\dfrac1{\tan y}
   

x=\dfrac12\pi+y

\begin{aligned}
\tan x&=\tan\left(\dfrac12\pi+y\right)\\
&=-\cot y\\
&=-\dfrac1{\tan y}
\end{aligned}

Jadi, \tan x=-\dfrac1{\tan y}.
JAWABB: E

---

No. 3

Jika \sin13\degree=a, maka nilai {\cot257\degree+\csc257\degree=}

1. \dfrac{a-1}{\sqrt{a^2-1}}
2. \dfrac{1-a}{\sqrt{a^2-1}}
   
3. \dfrac{a-1}{\sqrt{1-a^2}}
   

4. \dfrac{1-a}{\sqrt{1-a^2}}
5. \dfrac{-a-1}{\sqrt{1-a^2}}
   

\begin{aligned}
\cot257\degree+\csc257\degree&=\cot\left(270\degree-13\degree\right)+\csc\left(270\degree-13\degree\right)\\
&=\tan13\degree-\sec13\degree\\
&=\dfrac{a}{\sqrt{1-a^2}}-\dfrac1{\sqrt{1-a^2}}\\
&=\boxed{\boxed{\dfrac{a-1}{\sqrt{1-a^2}}}}
\end{aligned}

Jadi, \cot257\degree+\csc257\degree=\dfrac{a-1}{\sqrt{1-a^2}}.
JAWAB: C

---

No. 4

Diketahui \cos\alpha = \dfrac{a}{2b}, dengan \alpha sudut lancip dan b\neq0. Nilai dari \tan\alpha=

1. \dfrac{2b}a
2. \dfrac{\sqrt{a^2-4b^2}}{2a}
   
3. \dfrac{\sqrt{4b^2-a^2}}{2a}
   

4. \dfrac{\sqrt{a^2-4b^2}}a
5. \dfrac{\sqrt{4b^2-a^2}}a
   

\cos\alpha = \dfrac{a}{2b}=\dfrac{sa}{mi}
sa=a, mi=2b

\begin{aligned}
de&=\sqrt{(2b)^2-a^2}\\
&=\sqrt{4b^2-a^2}
\end{aligned}

\begin{aligned}
\tan\alpha&=\dfrac{de}{sa}\\
&=\boxed{\boxed{\dfrac{\sqrt{4b^2-a^2}}a}}
\end{aligned}

Jadi, \tan\alpha=\dfrac{\sqrt{4b^2-a^2}}a.
JAWAB: E

---

No. 5

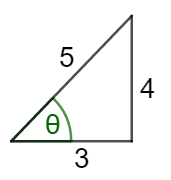
Jika \theta sudut lancip dan {\cos\theta=\dfrac35}, maka nilai dari \dfrac{\sin\theta\tan\theta-1}{2\tan^2\theta} adalah

Grup WhatsApp

\sin\theta=\dfrac45

\tan\theta=\dfrac43

\(\eqalign{ \dfrac{\sin\theta\tan\theta-1}{2\tan^2\theta}&=\dfrac{\left(\dfrac45\right)\left(\dfrac43\right)-1}{2\left(\dfrac43\right)^2}\\ &=\dfrac{\dfrac{16}{15}-1}{2\left(\dfrac{16}9\right)}\\ &=\dfrac{\dfrac1{15}}{\dfrac{32}9}\\ &=\dfrac1{15}\cdot\dfrac9{32}\\ &=\boxed{\boxed{\dfrac3{160}}} }\)

Jadi, \dfrac{\sin\theta\tan\theta-1}{2\tan^2\theta={\dfrac3{160}}.

Share this post