Berikut ini adalah kumpulan soal mengenai Turunan tingkat dasar. Jika ada jawaban yang salah, mohon dikoreksi melalui komentar. Terima kasih.
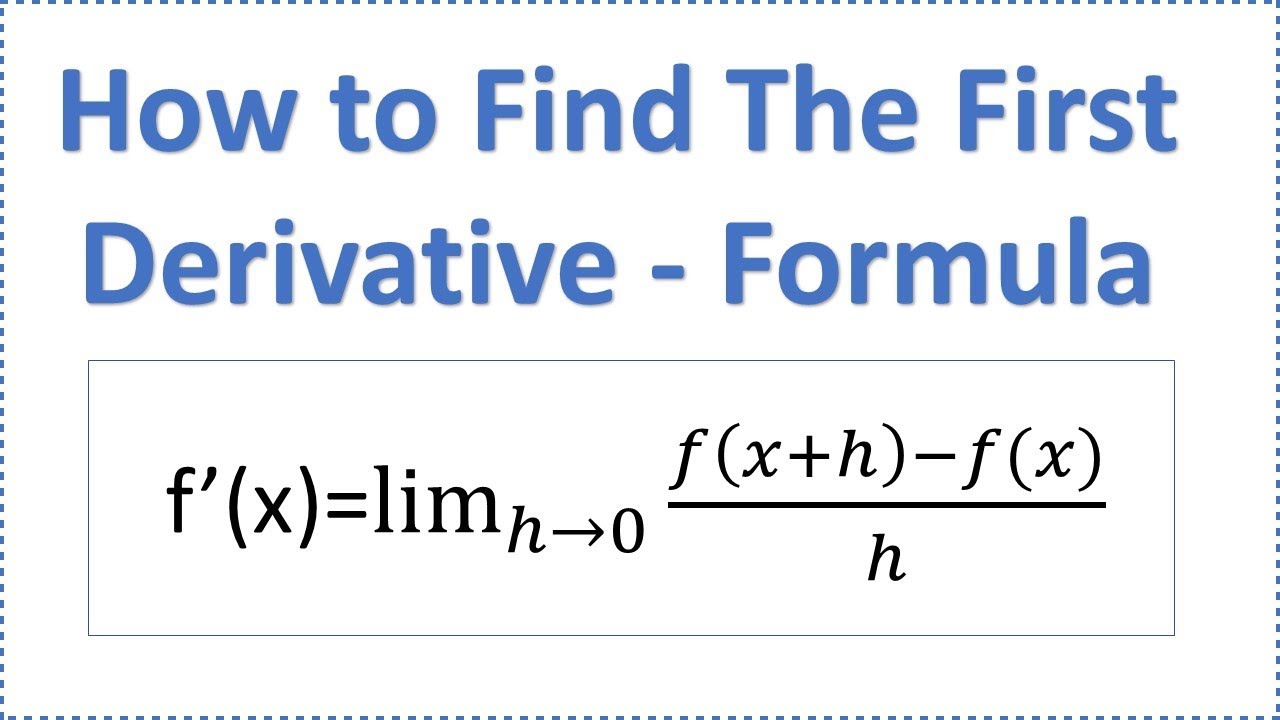
No. 1 Dengan menggunakan definisi turunan suatu fungsi, tentukan turunan pertama dari fungsi berikut.
f ( x ) = − 3 x 2 + 1 f(x)=\dfrac{-3}{x^2+1} f ( x ) = x 2 + 1 − 3 f ′ ( x ) = l i m h → 0 f ( x + h ) − f ( x ) h = l i m h → 0 − 3 ( x + h ) 2 + 1 −− 3 x 2 + 1 h = l i m h → 0 − 3 x 2 + 2 x h + h 2 + 1 + 3 x 2 + 1 h = l i m h → 0 − 3 ( x 2 + 1 ) + 3 ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) h = l i m h → 0 − 3 x 2 − 3 + 3 x 2 + 6 x h + 3 h 2 + 3 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = l i m h → 06 x h + 3 h 2 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = l i m h → 06 x + 3 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = 6 x + 3 ( 0 ) ( x 2 + 2 x ( 0 ) + ( 0 ) 2 + 1 ) ( x 2 + 1 ) = 6 x ( x 2 + 1 ) ( x 2 + 1 ) = 6 x ( x 2 + 1 ) 2 f′(x)=limh→0f(x+h)−f(x)h=limh→0−3(x+h)2+1−−3x2+1h=limh→0−3x2+2xh+h2+1+3x2+1h=limh→0−3(x2+1)+3(x2+2xh+h2+1)(x2+2xh+h2+1)(x2+1)h=limh→0−3x2−3+3x2+6xh+3h2+3h(x2+2xh+h2+1)(x2+1)=limh→06xh+3h2h(x2+2xh+h2+1)(x2+1)=limh→06x+3h(x2+2xh+h2+1)(x2+1)=6x+3(0)(x2+2x(0)+(0)2+1)(x2+1)=6x(x2+1)(x2+1)=6x(x2+1)2 f ′ ( x ) = l i m h → 0 f ( x + h ) − f ( x ) h = l i m h → 0 − 3 ( x + h ) 2 + 1 − − 3 x 2 + 1 h = l i m h → 0 − 3 x 2 + 2 x h + h 2 + 1 + 3 x 2 + 1 h = l i m h → 0 − 3 ( x 2 + 1 ) + 3 ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) h = l i m h → 0 − 3 x 2 − 3 + 3 x 2 + 6 x h + 3 h 2 + 3 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = l i m h → 0 6 x h + 3 h 2 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = l i m h → 0 6 x + 3 h ( x 2 + 2 x h + h 2 + 1 ) ( x 2 + 1 ) = 6 x + 3 ( 0 ) ( x 2 + 2 x ( 0 ) + ( 0 ) 2 + 1 ) ( x 2 + 1 ) = 6 x ( x 2 + 1 ) ( x 2 + 1 ) = 6 x ( x 2 + 1 ) 2 No. 2 Diketahui fungsi
f f f dan
g g g dengan
f ( x ) = x 2 − 2 x + 1 {f(x)=x^2-2x+1} f ( x ) = x 2 − 2 x + 1 dan
g ′ ( x ) = 10 − x 2 {g'(x)=\sqrt{10-x^2}} g ′ ( x ) = 1 0 − x 2 dengan
g ′ g' g ′ menyatakan turunan pertama fungsi
g ( x ) g(x) g ( x ) . Nilai turunan pertama fungsi
( g ∘ f ) ( x ) \left(g\circ f\right)(x) ( g ∘ f ) ( x ) untuk
x = 0 {x=0} x = 0 adalah ....
f ′ ( x ) = 2 x − 2 f'(x)=2x-2 f ′ ( x ) = 2 x − 2 ( g ∘ f ) ′ ( x ) = f ′ ( x ) ⋅ g ′ ( f ( x ) ) ( g ∘ f ) ′ ( 0 ) = f ′ ( 0 ) ⋅ g ′ ( f ( 0 ) ) = [ 2 ( 0 ) − 2 ] ⋅ g ′ ( 02 − 2 ( 0 ) + 1 ) = ( − 2 ) ⋅ g ′ ( 1 ) = ( − 2 ) 10 − 12 = − 29 = − 2 ⋅ 3 = − 6 (g∘f)′(x)=f′(x)⋅g′(f(x))(g∘f)′(0)=f′(0)⋅g′(f(0))=[2(0)−2]⋅g′(02−2(0)+1)=(−2)⋅g′(1)=(−2)10−12=−29=−2⋅3=−6 ( g ∘ f ) ′ ( x ) = f ′ ( x ) ⋅ g ′ ( f ( x ) ) ( g ∘ f ) ′ ( 0 ) = f ′ ( 0 ) ⋅ g ′ ( f ( 0 ) ) = [ 2 ( 0 ) − 2 ] ⋅ g ′ ( 0 2 − 2 ( 0 ) + 1 ) = ( − 2 ) ⋅ g ′ ( 1 ) = ( − 2 ) 1 0 − 1 2 = − 2 9 = − 2 ⋅ 3 = − 6 No. 3 Jika diketahui
f ( x ) = ( x 6 − 1 ) ( x 8 + 1 ) x 10 {f(x)=\dfrac{\left(x^6-1\right)\left(x^8+1\right)}{x^{10}}} f ( x ) = x 1 0 ( x 6 − 1 ) ( x 8 + 1 ) dan
f ′ ( x ) f'(x) f ′ ( x ) menyatakan turunan pertama
f ( x ) f(x) f ( x ) maka nilai
f ′ ( 1 ) f'(1) f ′ ( 1 ) adalah
f ( x ) = ( x 6 − 1 ) ( x 8 + 1 ) x 10 = x 14 + x 6 − x 8 − 1 x 10 = x 4 + x − 4 − x − 2 − x − 10 f ′ ( x ) = 4 x 3 − 4 x − 5 + 2 x − 3 + 10 x − 11 f ′ ( 1 ) = 4 ( 1 ) 3 − 4 ( 1 ) − 5 + 2 ( 1 ) − 3 + 10 ( 1 ) − 11 = 4 − 4 + 2 + 10 = 12 f(x)=(x6−1)(x8+1)x10=x14+x6−x8−1x10=x4+x−4−x−2−x−10f′(x)=4x3−4x−5+2x−3+10x−11f′(1)=4(1)3−4(1)−5+2(1)−3+10(1)−11=4−4+2+10=12 f ( x ) = ( x 6 − 1 ) ( x 8 + 1 ) x 1 0 = x 1 4 + x 6 − x 8 − 1 x 1 0 = x 4 + x − 4 − x − 2 − x − 1 0 f ′ ( x ) = 4 x 3 − 4 x − 5 + 2 x − 3 + 1 0 x − 1 1 f ′ ( 1 ) = 4 ( 1 ) 3 − 4 ( 1 ) − 5 + 2 ( 1 ) − 3 + 1 0 ( 1 ) − 1 1 = 4 − 4 + 2 + 1 0 = 1 2



0 Response to "Exercise Zone : Turunan (Derivative)"
Post a Comment